Facebook Youtube

Publications

Morphology of Surfaces in Mesoscopic Polymers, Surfactants, Electrons, or Reaction–Diffusion Systems: Methods, Simulations, and Measurements

A. Aksimentiev, M. Fiałkowski and R. Hołyst

Advances in Chemical Physics, 2002, 121, 141-239

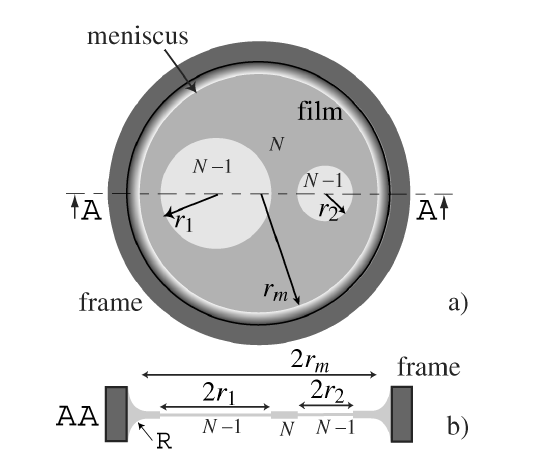
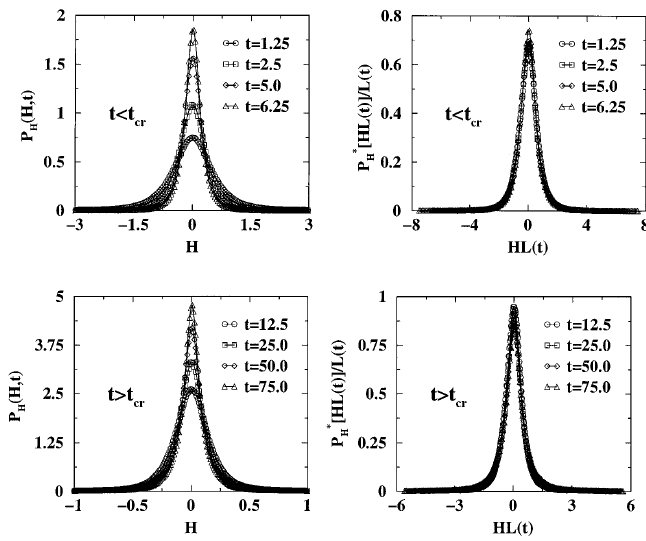
In this chapter the authors discuss systems with internal surfaces, ordered surfaces, topological transformations, and dynamical scaling. In Section II the authors show specific examples of mesoscopic systems with special attention devoted to the surfaces in the system—that is, periodic surfaces in surfactant systems, periodic surfaces in diblock copolymers, bicontinuous disordered interfaces in spinodally decomposing blends, ordered charge density wave patterns in electron liquids, and dissipative structures in reaction–diffusion systems. Section III covers the theory of morphological measures, including the Euler characteristic and the Gaussian and mean curvatures.

When Boundaries Dominate: Dislocation Dynamics in Smectic Films

P. Oswald, P. Pierański, F. Picano and R. Hołyst

Phys. Rev. Lett. 2002, 88, 015503

We discuss the influence of dissipation at a system boundary (film-meniscus interface) on the dynamics of dislocation loops inside a smectic film. This dissipation induces a strong coupling between dislocations—effectively independent of their separation—leading to their nontrivial dynamics. Because of these dynamics, the effective “dynamical” radius of nucleation can be 10 times larger than the usual static critical radius.

Contact Angle between Smectic Film and Its Meniscus

A. Poniewierski, P.Oswald and R. Hołyst

Langmuir 2002, 18, 5, 1511–1517

We apply de Gennes model (Langmuir, 1990, 6, 1448−1450) for presmectic ordering to a freely suspended smectic film coupled to the meniscus. We show that the quartic term in the expansion of the free energy in power of the smectic order parameter cannot be neglected in the model in order to explain the full behavior of the contact angle, θm, between the film and the meniscus. As shown previously (Phys. Rev. E2001, 63, 021705-1−021705-9), this contact angle is nonzero due to the disjoining pressure caused by the enhanced smectic ordering at the film surfaces. The temperature dependence of θm with a maximum above the bulk temperature of the nematic-smectic phase transition, TNA, is qualitatively reproduced by the model for all film thicknesses h = Nd, where N is the number of layers and d the smectic period, although some discrepancy between theory and experiment for the temperature at the maximum still remains. The apparent change of slope of the contact angle at TNA observed experimentally in thin films cannot be reproduced by the mean-field treatment of the model and presumably critical fluctuations in the meniscus and their influence on the behavior of the surface tension close to TNA have to be taken into account.

Annihilation of point defects on a line

R. Holyst and P. Oswald

Phys. Rev. E 2002, 65, 041711

We discuss the evolution of the distribution function of distances between point defects of opposite signs distributed on a line of finite length interacting via a potential attractive at short distances and repulsive at large distances. The standard deviation of the distribution grows quickly at short times, attains maximum, and decreases logarithmicaly at longer times. The distance between the defects increases monotonically and at equilibrium is about two times larger than the distance at which the repulsive force attains maximum. The distance dependent viscosity does not change qualitatively these conclusions, but only increases the time scale of evolution by one order of magnitude.

Scattering Patterns of Self-Assembled Cubic Phases. 2. Analysis of the Experimental Spectra

P. Garstecki and R. Hołyst

Langmuir 2002, 18, 7, 2529–2537

The method presented in the preceding article is applied in this paper to the analysis of X-ray spectra of cubic phases formed in the following systems:  1,2-dilauoryl-sn-glycero-3-phosphoethanolamine, 1,2-dielaidoyl-sn-glycero-3-phosphoethanolamine, 1,2-dioleoyl-sn-glycero-3-phosphocholine, and didodecyldimethylammonium bromide lipids in water; glycerolmonooleate amphiphilic molecules with Polaxamer P407 in water; R6FΣEO2 fluorinated surfactants in aqueous solutions and polimerized system formed in the cetyltrimethylammonium chloride with tetraethyl orthosilicate additives. We show that our analysis allows determination of the width of the layer decorating the periodic surface in the cubic phase, the composition of the cubic phase in the system with excess water, the type of the cubic phase (direct or inverse), surface area per head of the amphiphilic molecule, the volume fractions of the coexisting cubic phases, the epitaxial relations between the coexisting phases, and the kinetic pathways in the phase transitions between ordered phases.

Scattering patterns of self-assembled cubic phases. 1. The model

P. Garstecki and R. Hołyst

Langmuir 2002, 18, 7, 2519–2528

Bragg reflection amplitudes are examined for the structures P, D, G, C(P), C(D), I-WP, and F-RD in amphiphilic systems. For these ordered phases, a very simple analytical formula for the scattering amplitudes is given. The formula allows determination of the scattering amplitudes for any cross-sectional density profile of the membrane decorating the minimal surface. Within this approximation an analytical solution for the Debye−Waller factor is presented. Finally we propose a simplified model that can greatly facilitate examination of the experimental scattering patterns.

Morphology from the maximum entropy principle: Domains in a phase ordering system and a crack pattern in broken glass

M. Fiałkowski and R. Hołyst

Phys. Rev. E 2002, 65, 057105

The maximum entropy principle is applied to study the morphology of a phase ordering two-dimensional system below the critical point. The distribution of domain area A is a function of ratio of the area to contour length L, R=A/L(A), and is given by exp(−λRμ) with exponent μ=2, which follows from the Lifshitz-Cahn-Allen theory. A and L are linked through the relation L∼Aν. We find two types of domain in the system: large of elongated shape (ν=0.88) and small of circular shape (ν=0.5). A crack pattern in broken glass belongs to the same morphology class with μ=1 and ν=0.72.

Quench–jump sequence in phase separation in polymer blends

M. Fiałkowski and R. Hołyst

J. Chem. Phys. 2002, 117, 1886-1892

A two-step process of phase separation–mixing is analyzed for binary mixtures. The system is first quenched into the thermodynamical instability region (temperature T), where the mixture undergoes a process of spinodal decomposition, characterized for short times by the growth of the Cahn peak of a scattered intensity at fixed scattering wave vector. Next we heat up a system (make a temperature jump to temperature T1)T1) above the spinodal line (temperature Ts)Ts) and compute the decay of this peak. The peak intensity decreases and the peak position moves toward short wave vectors. The integrated peak intensity decreases exponentially at short times with a characteristic decay time that depends on T, T1,T1, and Ts.Ts. The increase of the Euler characteristic from large negative values toward zero suggests that the shift of the peak toward short wave vectors is associated with the disappearance of small connections in a bicontinuous structure formed in the early stages of spinodal decomposition. Slow decay of the surface area indicates that the domains keep their shape for a long time, despite the fast decay of the saturation of the concentration field inside them.

Memory Effects in Homopolymer Blends during Annealing

M. Graca, S. A. Wieczorek, and R. Hołyst

Macromolecules 2002, 35, 20, 7718–7724

We perform light scattering and direct optical experiments on a homopolymer blend of poly(methylphenylsiloxane) (PMPS) with polystyrene (PS). The system is subjected to the three-step process. The system is first quench to low temperature (T1) and allowed to separate for 5 h; next it is heated to a high temperature (T0) to the one-phase region where it mixes for a couple of minutes (1−10 min) and then quenched back to T1 and observed for 5 h. We note that annealing at T0 can be quantitatively studied by the analysis of the scattering intensity summed over a linear array of photodiodes. This quantity is very sensitive to the structure exisiting in the system. If the system is properly annealed, it has a noisy behavior and while the structure inside the system persists, it behaves very regularly. Moreover, one can observe the differences in the scattering intensity between the first and the second quench at very short wavevectors, indicating that large domains survived the annealing process for short annealing time (less than 4 min). However, the average area of the domains per unit volume is the same as obtained from the tail of the scattering intensity, indicating that small domains dominating in the system do not survive the mixing process even if it is very short (2 min). Finally, the direct observation under the microscope reveals that they dissolve in such a way that their size changes at the end of the process of dissolution, when as we suspect the size of the interface becomes comparable to the size of the dissolving domain. Domains inside the domains are also observed at short times after the second quench. In general, our methods allow the quantitative estimate of the annealing time for polymer mixtures and thus can save a lot of time, especially if we have to repeat the same measurements many times and we need to anneal the samples between measurements.

Morphological changes during the order-disorder transition in the two- and three-dimensional systems of scalar nonconserved order parameters

M. Fiałkowski and R. Hołyst

Phys. Rev. E 2002, 66, 046121

The order-disorder transition is studied in a system of a scalar nonconserved order parameter. We use this well studied system to show that the application of the methods of topology and geometry reveals that our knowledge of the kinetic pathways by which the order-disorder transition proceeds is far from being complete. We show that in two-dimensional (2D) and 3D systems there are three dynamical regimes in the evolution of the system: early, intermediate, and late. In the intermediate regime two length scales govern the behavior of the system, whereas in the early and intermediate regime there is only one length scale. The size distribution of the domain area indicates the pathway by which the domains change their size. There are only two types of domains in a 2D system: circular and elongated with well defined characteristics (scaling of the area with the contour length) which in the late regime do not depend on time after rescaling by the average area and contour in the system. The elongated domains continuously change into circular domains reducing in this way the overall dissipation in the system. In order to reach a Lifshitz-Cahn-Allen (LCA) late stage regime the number of elongated domains must be strongly reduced. In the intermediate regime the number of elongated domains is large and simple LCA scaling does not hold. In a 3D symmetric system we always have a bicontinuous structure that evolves by cutting small connections. The late stage regime seems to be associated with the appearance of the preferred nonzero mean curvature. The early-intermediate regime crossover is associated with the saturation of the order parameter inside the domains, while the intermediate-late stage regime crossover is related to the global breaking of the ± order parameter symmetry (marked by the appearance of the nonzero mean curvature but still zero average magnetization). The times for the occurrence of these crossovers do not depend on the size of the system.

Demixing/mixing of polystyrene, with poly(methylphenylsiloxane) in a two-step cooling/heating process: Jump spinodal specification method

M. Graca, S. A. Wieczorek, M. Fiałkowski, and R. Hołyst

Macromolecules 2002, 35, 24, 9117–9129

We present experimental studies of the mixing process of a homopolymer blend of poly(methylphenylsiloxane) (PMPS) with polystyrene (PS). The system is first allowed to decompose spinodally at low temperature for few minutes and next is heated to a higher temperature to the one-phase or two-phase (metastable or unstable) region. In all cases the intensity drops initially after the temperature jump. In the one-phase region the intensity drops to the base scattering. In the metastable region it drops initially, and later on it starts to grow. In this region the peak position shifts strongly toward small wavevectors, and the intensity drops considerably. Finally, in the spinodal (unstable) region the peak position shifts toward smaller wavevectors, but the intensity of the peak hardly changes before increasing again. The decrease of the peak intensity is exponential with the characteristic decay time which approaches infinity when the temperature of the jump approaches that of the quench. The mixing process mainly involves the interdiffusion of polymers, without global movement of the interface. Small domains disappear faster than larger domains, and therefore the peak position (indicating an average size of the domains) shifts toward smaller wavevectors. In the metastable region the average wavevector as a function of time has a characteristic minimum, which shifts toward zero as we approach the spinodal. If the jump is made to the unstable region, the average wavevector monotonically decreases with time. The average wavevector, after temperature jump, allows to predict the location of the binodal and spinodal. We call this new method of spinodal location a jump spinodal specification method (JSS method). The generic features of this method have been confirmed in the computer simulations of the Flory−Huggins−de Gennes model with the Langevin dynamics for the mixture of polybutadiene and deuterated polybutadiene.

Photonic properties of multicontinuous cubic phases

V. Babin, P. Garstecki and R. Hołyst

Phys. Rev. B 2002, 66, 235120

We present a systematic study of the photonic properties (band structures) of periodic multicontinuous cubic phases based on the P, D, G, I−WP, F−RD, and C(P) triply periodic minimal surfaces. We investigate the structures with up to five separate interwoven subvolumes. The influence of the dielectric constant modulation at different spatial scales is discussed. The lowest dielectric constant contrasts required to observe the full three-dimensional photonic band gaps are stated.

Application of the Euler characteristic to the study of homopolymer blends and copolymer melts

A. Aksimentiev and R. Hołyst

POLIMERY 2001, 6, 5, 307-323

Scaling of the Euler Characteristic, Surface Area, and Curvatures in the Phase Separating or Ordering Systems

M. Fiałkowski, A. Aksimentiev and R. Hołyst

Phys. Rev. Lett. 2001, 86, 240

We present robust scaling laws for the Euler characteristic and curvatures applicable to any symmetric system undergoing phase separating or ordering kinetics. We apply it to the phase ordering in a system of the nonconserved scalar order parameter and find three scaling regimes. The appearance of the preferred nonzero curvature of an interface separating ± domains marks the crossover to the late stage regime characterized by the Lifshitz-Cahn-Allen scaling.

Liquid‐Crystalline Order in Polymer Systems: Basic Models

R. Hołyst and P. Oswald

Macromolecular Theory and Simulations, 2001, 10, 1, 1-16

The liquid crystalline (LC) order appears in a variety of polymer systems, such as solutions of rod‐like molecules (DNA, TMV), solutions of semiflexible molecules (long fragments of DNA), block‐copolymer melts, main‐chain and side‐chain liquid crystalline polymer melts etc. Many LC phases have been observed in these systems; the most common being: the nematic, cholesteric, smectic or lamellar, hexagonal, and double gyroid (in block copolymers) phases. We will discuss in detail some of them and give their quantitative description in terms of order parameters. We will also present various theoretical models used to study LC ordering in the systems. The models discussed in this paper are as follows: Onsager model and its extension within the Density Functional Theories (DFT), Khohlov‐Semenov model for semiflexible polymers, Kratky‐Porod model, combination of the Kratky‐Porod model and Maier‐Saupe model, self‐consistent field theoretical model and finally the Landau‐Ginzburg models and their connection with the Edwards model for polymer systems. We will also briefly discuss the elasticity of polymer systems in the case of nematic ordering. We present in a pedagogical manner the general ideas which are behind various models and give references to the papers which contain the technical details.

(+48) 22 343-31-23

softmatter@ichf.edu.pl

Kasprzaka 44/52, 01-224 Warsaw, Poland