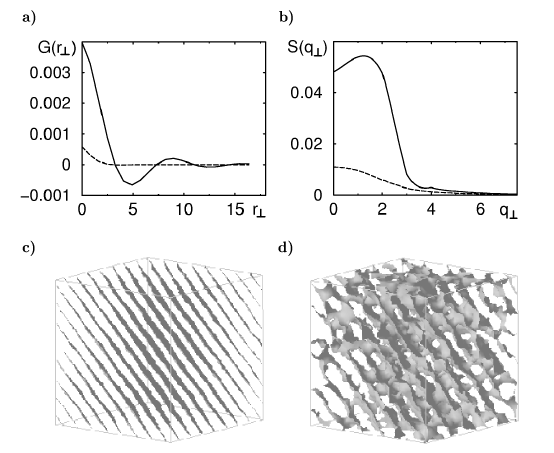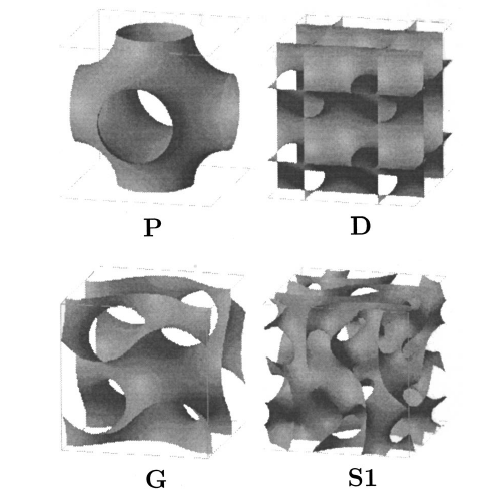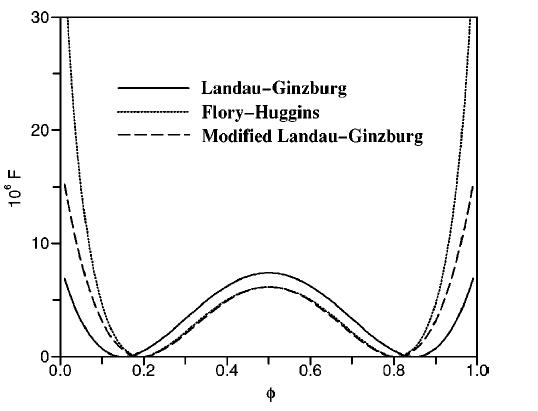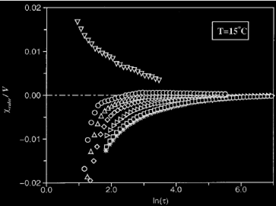
Entropy-driven phase transitions and the Kauzmann entropy crisis
R. Hołyst
Physica A: Statistical Mechanics and its Applications 2001, 292, 1–4, 255-258
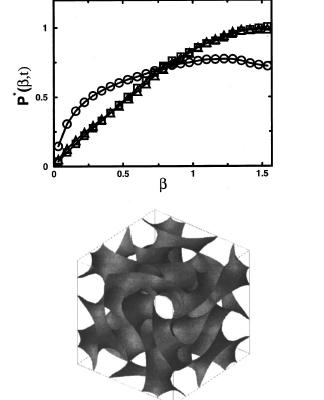
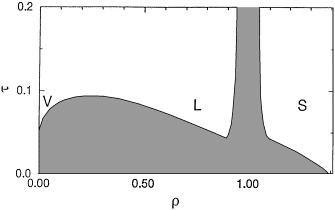
We briefly present the Kauzmann entropy crisis and show that it may have a simple explanation in terms of the entropy-driven phase transitions. We discuss in a pedagogical manner why the enthalpy change at the freezing transition is positive even for a hard sphere fluid, despite the fact that the transition is driven by the increase of the entropy. We use this observation to explain qualitatively the Kauzmann entropy crisis.